mratio Class Reference
Class representing ratio of two densities which arise e.g. by applying the Bayes rule. It represents density in the form:
![\[ f(rv|rvc) = \frac{f(rv,rvc)}{f(rvc)} \]](form_79.png)
where  .
More...
.
More...
#include <emix.h>


Public Member Functions | |
| mratio (const epdf *nom0, const RV &rv, bool copy=false) | |
| double | evallogcond (const vec &val, const vec &cond) |
| Shortcut for conditioning and evaluation of the internal epdf. In some cases, this operation can be implemented efficiently. | |
| void | ownnom () |
| Object takes ownership of nom and will destroy it. | |
| ~mratio () | |
| Default destructor. | |
| virtual vec | samplecond (const vec &cond, double &ll) |
| Returns the required moment of the epdf. | |
| virtual mat | samplecond_m (const vec &cond, vec &ll, int N) |
| Returns. | |
| virtual void | condition (const vec &cond) |
Update ep so that it represents this mpdf conditioned on rvc = cond. | |
| virtual vec | evallogcond_m (const mat &Dt, const vec &cond) |
| Matrix version of evallogcond. | |
| RV | _rvc () const |
| access function | |
| RV | _rv () const |
| access function | |
| epdf & | _epdf () |
| access function | |
Protected Attributes | |
| const epdf * | nom |
| Nominator in the form of mpdf. | |
| epdf * | den |
| Denominator in the form of epdf. | |
| bool | destroynom |
| flag for destructor | |
| datalink_m2e | dl |
| datalink between conditional and nom | |
| RV | rv |
| modeled random variable | |
| RV | rvc |
| random variable in condition | |
| epdf * | ep |
| pointer to internal epdf | |
Detailed Description
Class representing ratio of two densities which arise e.g. by applying the Bayes rule. It represents density in the form:
![\[ f(rv|rvc) = \frac{f(rv,rvc)}{f(rvc)} \]](form_79.png)
where  .
.
In particular this type of arise by conditioning of a mixture model.
At present the only supported operation is evallogcond().
Constructor & Destructor Documentation
Default constructor. By default, the given epdf is not copied! It is assumed that this function will be used only temporarily.
References den, destroynom, RV::length(), epdf::marginal(), nom, and mpdf::rvc.
Member Function Documentation
| virtual vec mpdf::samplecond | ( | const vec & | cond, | |
| double & | ll | |||
| ) | [inline, virtual, inherited] |
Returns the required moment of the epdf.
Returns a sample from the density conditioned on cond, 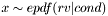 .
.
- Parameters:
-
cond is numeric value of rvll is a return value of log-likelihood of the sample.
Reimplemented in mprod.
References mpdf::condition(), mpdf::ep, epdf::evallog(), and epdf::sample().
Referenced by MPF< BM_T >::bayes(), and PF::bayes().
| virtual mat mpdf::samplecond_m | ( | const vec & | cond, | |
| vec & | ll, | |||
| int | N | |||
| ) | [inline, virtual, inherited] |
Returns.
- Parameters:
-
N samples from the density conditioned on cond,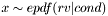 .
. cond is numeric value of rvll is a return value of log-likelihood of the sample.
References mpdf::condition(), RV::count(), mpdf::ep, epdf::evallog(), mpdf::rv, and epdf::sample().
The documentation for this class was generated from the following file:
- work/git/mixpp/bdm/stat/emix.h
 1.5.6
1.5.6