bdm::mlognorm Class Reference
Log-Normal random walk. More...
<<<<<<< HEAD:library/doc/html/classbdm_1_1mlognorm.html
#include <exp_family.h>
=======
#include <exp_family.h>
>>>>>>> doc:library/doc/html/classbdm_1_1mlognorm.html
Public Member Functions | |
| mlognorm () | |
| Constructor. | |
| void | set_parameters (int size, double k) |
Set value of k. | |
| void | condition (const vec &val) |
Update ep so that it represents this mpdf conditioned on rvc = cond. | |
| void | from_setting (const Setting &set) |
| virtual string | to_string () |
| This method returns a basic info about the current instance. | |
| virtual void | to_setting (Setting &set) const |
| This method save all the instance properties into the Setting structure. | |
| virtual void | validate () |
| This method TODO. | |
Matematical operations | |
| virtual vec | samplecond (const vec &cond) |
Returns a sample from the density conditioned on cond, 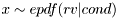 . . | |
| virtual mat | samplecond_m (const vec &cond, int N) |
| Returns. | |
| virtual double | evallogcond (const vec &dt, const vec &cond) |
| Shortcut for conditioning and evaluation of the internal epdf. In some cases, this operation can be implemented efficiently. | |
| virtual vec | evallogcond_m (const mat &Dt, const vec &cond) |
| Matrix version of evallogcond. | |
| virtual vec | evallogcond_m (const Array< vec > &Dt, const vec &cond) |
| Array<vec> version of evallogcond. | |
Access to attributes | |
| RV | _rv () |
| RV | _rvc () |
| int | dimension () |
| int | dimensionc () |
| epdf & | _epdf () |
| epdf * | _e () |
Connection to other objects | |
| void | set_rvc (const RV &rvc0) |
| void | set_rv (const RV &rv0) |
| bool | isnamed () |
Protected Attributes | |
| elognorm | eno |
| double | sig2 |
| parameter 1/2*sigma^2 | |
| vec & | mu |
| access | |
| int | dimc |
| dimension of the condition | |
| RV | rvc |
| random variable in condition | |
| epdf * | ep |
| pointer to internal epdf | |
Detailed Description
Log-Normal random walk.
<<<<<<< HEAD:library/doc/html/classbdm_1_1mlognorm.html
Mean value,  , is...
, is...
==== Check == vv = Standard deviation of the random walk is proportional to one 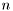 -th the mean. This is achieved by setting
-th the mean. This is achieved by setting  and
and 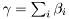 .
.
The standard deviation of the walk is then:  .
.
Member Function Documentation
======= Mean value,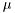 , is...
, is...
==== Check == vv = Standard deviation of the random walk is proportional to one 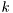 -th the mean. This is achieved by setting
-th the mean. This is achieved by setting 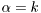 and
and 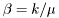 .
.
The standard deviation of the walk is then: 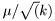 .
.
Member Function Documentation
>>>>>>> doc:library/doc/html/classbdm_1_1mlognorm.html| void bdm::mlognorm::from_setting | ( | const Setting & | set | ) | [virtual] |
The mlognorm is constructed from a structure with fields:
system = {
type = "mlognorm";
k = 0.1; // constant k
mu0 = [1., 1.];
// == OPTIONAL ==
// description of y variables
y = {type="rv"; names=["y", "u"];};
// description of u variable
u = {type="rv"; names=[];}
};
Reimplemented from bdm::mpdf.
<<<<<<< HEAD:library/doc/html/classbdm_1_1mlognorm.htmlReferences condition(), bdm::UI::get(), and set_parameters().
=======References condition(), and set_parameters().
>>>>>>> doc:library/doc/html/classbdm_1_1mlognorm.html| virtual vec bdm::mpdf::samplecond | ( | const vec & | cond | ) | [inline, virtual, inherited] |
Returns a sample from the density conditioned on cond, 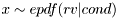 .
.
- Parameters:
-
cond is numeric value of rv
Reimplemented in bdm::mprod.
<<<<<<< HEAD:library/doc/html/classbdm_1_1mlognorm.htmlReferences bdm::mpdf::condition(), bdm::mpdf::ep, and bdm::epdf::sample().
Referenced by bdm::MPF< BM_T >::bayes(), bdm::PF::bayes(), and bdm::ArxDS::step().
=======References bdm::mpdf::condition(), bdm::mpdf::ep, and bdm::epdf::sample().
Referenced by bdm::MPF< BM_T >::bayes(), bdm::PF::bayes(), and bdm::ArxDS::step().
>>>>>>> doc:library/doc/html/classbdm_1_1mlognorm.html| virtual mat bdm::mpdf::samplecond_m | ( | const vec & | cond, | |
| int | N | |||
| ) | [inline, virtual, inherited] |
Returns.
- Parameters:
-
N samples from the density conditioned on cond,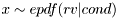 .
. cond is numeric value of rv
References bdm::mpdf::condition(), bdm::epdf::dimension(), bdm::mpdf::ep, and bdm::epdf::sample().
=======References bdm::mpdf::condition(), bdm::epdf::dimension(), bdm::mpdf::ep, and bdm::epdf::sample().
>>>>>>> doc:library/doc/html/classbdm_1_1mlognorm.html
The documentation for this class was generated from the following files:
-
<<<<<<< HEAD:library/doc/html/classbdm_1_1mlognorm.html
- exp_family.h
- exp_family.cpp
